Assessment: Notebook and Multiple-Choice Quizzes

Written by: Dr. Julian Fleron

Notebook Quizzes

In my blog on the assessment of Student Solutions and Proofs I described how I assess student work on fine prompts when students craft solutions/proofs for a chapter of work. This is an important opportunity for them to practice their writing. It also helps them review their understanding of the mathematics at hand. Both of these things are valuable. However, with a frequency approaching once every two weeks, it imposes a significant workload on the students as such a write-up may take three or four hours to complete. Moreover, it begins to feel monotonous to students if it is the only form of assessment.

To balance things I alternate full chapter write-ups with “notebook quizzes.” Click the thumbnail images to see examples of graded notebook quizzes. 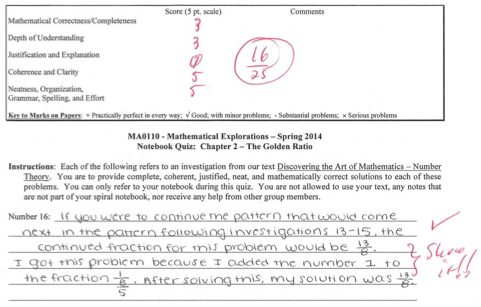Students are required to keep all of their coursework in spiral bound notebooks. When I feel we have had sufficient time on a chapter, I announce a notebook quiz for the next class. When students arrive they are given a double-sided sheet of paper with 8 – 10 investigations indicated by number only and blank spaces to record their answers. Students are not allowed to have the chapters available, only their work referenced by investigation numbers in their notebooks. The students do not explicitly know what the questions are (so they cannot attempt to solve them on the fly), they have only their original work in their notebook to craft suitable explanations. Experience has shown this to be a reasonable means of assessment. It cuts down slightly on grading time (fewer pages to flip around). Moreover, it instills in students the importance of organizing their work, articulating their solutions in their notebooks, and keeping current with the pace of the class.

Multiple Choice Notebook Quizzes

I have experimented with multiple choice notebook quizzes. For fairly routine investigations it works well – it is easy to create incorrect solutions based on typical misperceptions. However, for less routine investigations it is harder to craft solutions that really check understanding appropriately. Also problematic is the importance of explanations/proofs, something one cannot mimic very well on a multiple choice instrument. Finally, the use of inquiry-based learning routinely illustrates that students have widely varied correct approaches to solve a given problem. With a multiple choice instrument the carefully crafted correct solution may be unintelligible to students who have taken a completely different, albeit perfectly legitimate, approach.

Oral Quizzes

I have experimented with oral quizzes for students. For small, well-defined tasks (e.g. proofs of checkerboard tiling problems or mathematical magic tricks) this works well. But for larger, more diverse collections of investigations this is quite difficult. It takes an enormous amount of time to meet with all of the students and I do not find my own standards to be consistent across a number of days, times, and contexts.