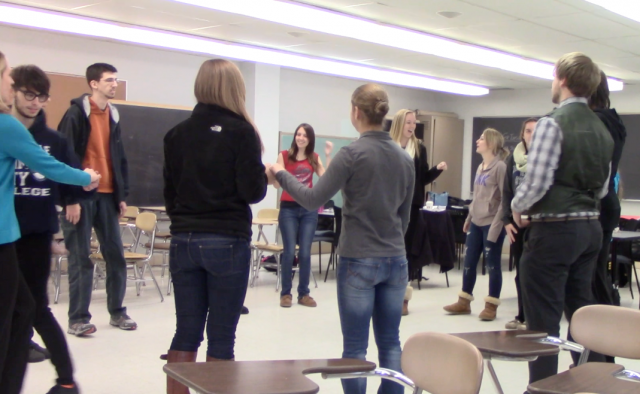Salsa Rueda and Mathematics

Written by: Dr. Christine von Renesse.

Instead of describing a particular teaching technique, this (shorter) blog will expose you to many ideas that come up for me around teaching a specific topic, salsa rueda, in a math for liberal arts class. I will tell you why I love to include dancing in my math classes and show you videos and student work from my math and dance class. Maybe you also want to give it a try some day?

Dancing...

One of my favorite activities in our dance book is dancing salsa rueda with my students. We know that movement can be very helpful in learning -- for various reasons (Jensen, 2000). For me, the movement motivates the mathematical questions but it also helps to engage all students, bring lightness and laughter into the classroom and build a strong classroom community. The first time moving together can be scary (for the students and the instructor). Many students have shared with me that they are as afraid of dancing as they are of mathematics! But the dancing we do is really about moving, with no attachment to “doing it perfectly”. Salsa teacher Daniel Trenner has developed some wonderful starting exercises that are easy and fun and help build the foundations for dancing salsa.

I will always remember dancing the salsa rueda, and laughing about how I can’t dance. I loved this class, even though it was hard. ...I know I didn’t do so hot on the proofs, but I had a lot of fun trying them, and I made some really good friends through it.
[DAoM Student, Fall 2013]

The exact steps at the right time is not as important as getting to where you are supposed to be, similarly to how arithmetic is not as important in my class as understanding the big idea. In many ways, it is easier to model in dance how we can laugh when something didn’t work out and how we can collaborate on a given task. And I believe that these are the messages students need to understand if they want to learn mathematics well. (Kapur, 2011)

In Salsa Rueda, pairs of dancers stand in a circle and dance the same salsa moves simultaneously. There is a leader of the circle who calls out the moves and everyone needs to listen and react in time. Each pair has a leader and a follower, which students determine by preference and confidence (instead of gender). As you can imagine, the frequent chaos often leads to laughter and “redo’s”.

I invite you to watch the following video clip, which shows students dance salsa rueda in my Math Explorations class in Fall 2013:

The Question:

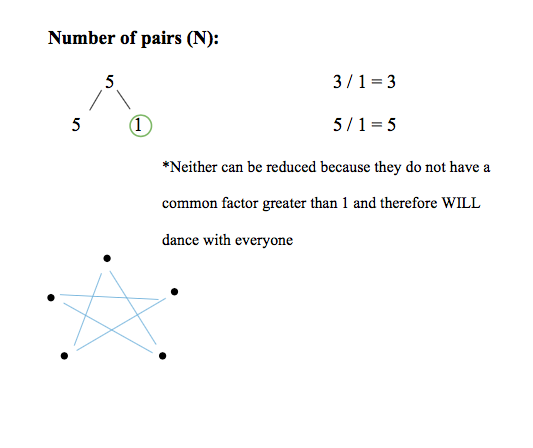
The main mathematical question is quite simple: There is a move called “dame” in which each leader passes their follower to the next leader in the circle and gets in turn a new follower from the other side. This can be done skipping one or several leaders, leading to an interesting pattern. In fact, what you get are star polygons!

In the language of the dance we are wondering:
1. Does each follower return eventually to his or her original leader? Does the answer depend on the number of dancers?
2. Does every follower get to dance with every leader in the circle? Does the answer depend on the number of dancers?
3. How many times does the follower move around the circle before they return to their original leader (if they do)?
My students spent several class periods looking for patterns, creating conjectures, and trying to make sense of why these conjectures are true. At various points I am using whole class discussions to let my students build on their classmate’s ideas.

I invite you again to watch a video clip from my class in which a student presents her work on question (2), by describing the patterns and conjectures that she found. I like how she explains her process and how open she is about what she doesn’t know (yet). In the image you can see her table where she recorded the number of partners (P) and the number of dame’s (D). The “NO” table records all examples where followers don’t dance with every leader, the “YES” table all others.

Connections...

One of the key ideas in making sense of the salsa rueda conjectures are common factors. In the next video clip you can see how a week later, in the same classroom, I am recording students’ conjectures about relating the gcf and the lcm. I show this clip often at professional development workshops because you can see how I record ideas that are mathematically incorrect or irrelevant without losing my “poker face” (see also my making mistakes blog).

Salsa rueda has other intriguing connections to general star polygons, the dance of the planets, rotational symmetry and spirographs.

From Investigations to Proofs

It is a difficult task to invent investigations that are “just right” for our students. In the creating inquiry activities blog (paper forthcoming in PRIMUS), Professor Mairead Greene and I describe the processes we use to fine-tune our tasks. The investigations lead the students to make conjectures and eventually prove them. The proof doesn’t have to be formal, but it has to establish an explanation that helps us make sense of why the conjecture is always true (see Proof as Sense Making ). I like the class to take turns between thinking about the mathematics and returning to the movement to test their conjectures or just give our brains a break. Students need to consider the gcf and the lcm to state their conjectures and their sense making often includes models like the number line, factor tress and star polygons. Here is a typical student work form my mathematics for liberal arts class: student work .

You can see that the student is still missing a complete deductive argument for the second conjecture, probably not yet understanding that there is a level of explanation missing. I like to use peer review to help find these "holes" and improve the work.

I remember Professor Von saying in class that she could tell who wrote which proof without looking at the name because we used a specific tone and our unique voice to write them. Initially, I did not believe her because I thought our voice only came out in English writing assignments. But then I realized that proofs are writing assignments. We explain our argument, come up with a conjecture and prove it using what we know and what we are learning, just like in Composition. ... I found that further into the semester I began challenging myself more and becoming more accepting of feedback from peers and professors. I became comfortable crossing out entire ideas and sentences and when we had discussions in class about how to solve the Salsa partner proof; I gave my input and listened to other groups’ ideas. I found that their ideas sometimes helped me get closer to proving my conjecture.
[DAoM Student, Fall 2013]

The salsa rueda unit can easily take a few weeks and can be followed by diving deeper into partner salsa dancing. But in my experience students like to change the topic of the class after a few weeks, and so I often let them choose the next topic from the variety of our books. Please feel free to email me if you have questions or thoughts about dancing and mathematics.

References:

Jensen, E. (2000). Moving with the Brain in Mind. Educational Leadership, 58(3), 34.

Kapur, Manu. "A further Study of Productive Failure in Mathematical Problem Solving: Unpacking the Design Components." Instructional Science 39.4 (2011): 561-79. ProQuest. Web. 6 Mar. 2016.

The Salsa Rueda Wiki is a great reference to learn all the different moves in salsa rueda.

Karl Schaffer and Erik Stern at MathDance are a wonderful resource for math and dance connections and performances. They visited my class in 2013 and let my students explore rope polyhedra.