A Path to Creating Inquiry Activities

Written by: Dr. Christine von Renesse

Often people ask me how to write inquiry activities so there must be some tricks that can be communicated. I don’t think that there is one way of writing “perfect” tasks for an inquiry-based classroom. In fact even in our project of just 4 people we write tasks very differently. Together with Prof. Mairead Greene from Rockhurst University, I have been thinking about the questions we ask ourselves before, during and after writing tasks (we are hoping for future publication in PRIMUS, focusing on IBL activities for calculus). Our intention is that these questions will help to inspire and guide others in creating good IBL activities in any mathematics course. In this blog I use “we” to represent the joint work with Mairead Greene and we use the terms task/activity as a synonym for problem, question, investigation, exploration, etc. This blog is very long so feel free to skip some of the the explanations and just find the questions in the gray boxes below. :)

Bigger Picture

It is easy for me to get lost in details and forget the main goals that drive my course. But there are many “big picture” questions we like to consider before starting to write activities.

We invite you to answer the questions in this blog while thinking about a specific course for which you would like to create an activity.

What kind of IBL do I want to use?

You can read the IBL blog to see how we define IBL. How you plan to use your activities in your classroom has of course an impact on how you write them. Inquiry is used in a wide variety of ways in quite varying classrooms. It can range from almost entirely presentation style with strictly individual work only (for instance using the Moore Method ) to almost entirely group work . While the IBL style hugely influences how you run your classroom, you might be able to use the same activities for any kind of IBL class. So we hope that our guiding questions will be helpful for any choice of IBL.

What is special about my Audience?

Of course audience also plays a large role in how we write our tasks. I have for example different priorities of my “meta goals” ( See Students Goals ) depending on the audience. In my math for liberal art class, it is most important for me that students get a positive attitude towards doing mathematics. In my calculus classes I first of all want my students to become independent and creative problem solvers and in my pre-service teacher classes (for elementary school) I want my students to be less anxious about mathematics and start believing in their ability to make sense of mathematics. Here are some questions that help us explore our meta goals:

• Are my students interested in the mathematics that I love or do they actually prefer a plug-and-chuck mathematics version from high school?
• Have they ever thought about proofs and what mathematics really is about?
• Are my students already confident, curious, motivated, persistent, willing to make mistakes, share their thinking, and change their thinking?
• Have my students worked in groups before?
• What exactly are my meta goals for this class? How do I prioritize them?
• How much time and effort am I willing to spend on working towards 
these meta-goals?
• Do I want to tailor my tasks and assessments such that students will also consciously work on the above meta-goals?

IBL lends itself beautifully to differentiated instruction . We like to ask ourselves:

• What is the minimal knowledge I expect all my students to achieve?
• Do I want the stronger students to get much farther and deeper than my weaker students? How much effort am I willing to spend 
on creating valuable extension questions?
• How difficult can I make the tasks so that most of my students are working at their learning edge (being neither bored nor too frustrated)? How much do my students know already and how fast do they learn?

Representations, Skills and Conceptual Understanding

When creating activities we have a few guiding principles that are seen in everything we write. Firstly, we write tasks that require students to work with a concept or object in more than one representation. The representations we typically use are symbolic, graphical, numerical and narrative although we sometimes also use a physical model to help students make connections. Transferring understanding developed in one representation and applying it to a new representation helps students to develop an understanding of a concept [9] and not just a method for working in a particular situation. In addition, understanding a concept in multiple representations helps students develop a more abstract understanding of that concept.

In the following video clip you can watch students share their representations for dance positions in reflective symmetry. Notice that the students invented the representations themselves which allows them to participate in an important part of mathematics that is often only provided for them.

In most areas of mathematics we want students to be able to competently develop and use certain skills/facts and methods while also developing a deep understanding of the relevant concepts. (I say “most” here, since in mathematics for liberal art courses I don’t focus on students memorizing skills and methods. The course is not a requirement for any other course and a deep conceptual understanding of some aspect of mathematics is my main goal.) For the purposes of most classes though, we think about facts as something that the student has memorized whether it be a definition, theorem or even a proof of a theorem if appropriate. There is no understanding of concepts necessary for this although students may indeed understand some or all of what is behind what they are memorizing. We use the term skills to describe one-step processes that the students learn to complete. This is somewhat course dependent as it depends on the student abilities. For instance in most calculus classes we would assume that calculating the average rate of change is a one-step process for students whereas the same may not be true in an algebra class. Methods are multi-step processes that the student will apply repeatedly. For instance, in calculus 1, applying the chain rule or product rule would be considered a method as would estimating a limit numerically. Finally, we want students to develop conceptual understanding, which we categorize as anything that does not fall into the skills/facts or methods category. More detail can be found in Building Conceptual Understanding in Precalculus .

Writing Inquiry Tasks

Questions to ask before the Writing Phase

What is the mathematical idea that I would like students to understand? What are the skills, methods and concepts necessary for or developed as part of this mathematical idea? What representations are there available for this mathematical idea?

Some examples of mathematical ideas from my classes are:

• The derivative of a function at a point is the limit of the average rates of change of f as the distance of the x-values gets closer to zero. (calculus 1)
• Triangles on a sphere behave differently than triangles in the plane. They don’t have an angle sum of 180 degrees. (modern geometries)
• Shifting the x value of a function by a positive value k forces the graph to move to the left by k, since f(x+k) is the value that you get by moving k to the right and then taking the y-value. (algebra)


For the last mathematical idea, an important skill would be to understand the function notation f(x), a method would be do be able to draw the graph of a simple (linear, quadratic) function. An example of conceptual understanding would be to connect how plugging in different values for k effects the y-values and hence the position of the graph.



What do my students not understand when I currently teach this topic? What are the subtle points that I would like them to understand but that they might miss?

This is where the mathematical knowledge of teaching (MKT) really comes in. Deborah Ball has thought a lot about MKT on the elementary school level and I believe we need to think about it more in higher education mathematics as well. In my experience, just because I know some topic in mathematics really well doesn’t mean that I know how to teach it well. Can I for instance predict the misconceptions students will have? It is powerful when we can find a task where an incorrect answer will highlight exactly the subtle point, misconception or mistake that we are interested in.
When Julian Fleron and I jointly taught a mathematics for liberal arts class we realized for example that students did not visualize tangent lines in the way we predicted. See image below. In our calculus book students are now encouraged to explore tangent lines physically first to get a deeper understanding of their properties.






What do students currently know that can be a gateway to this idea/concept? What can motivate this concept and give it some concrete meaning for the students?

Introducing a concept within a context allows students to investigate the unfamiliar concept in a familiar environment. Here are some examples of story contexts that I like:

• Linear equations by recording one or several runner’s position over time. Suddenly the intercepts, slopes and such have nice meanings. See activity.
• Derivative definition by computing the speed of a car at a specific point in time. See activity at iblcalculus.com.
• Reflectional and rotational symmetry by moving in different kinds of symmetry with a partner. See dance book
• Directional derivative definition by imagining a bug crawling through a room of varying temperature. Calculus 3 materials

Questions during the writing phase:

Can I create a need or a conflict that lets the students want to discover the answer and find a resolution?

Creating questions that make students curious so that they actually want to answer them is a great way to begin an activity. We like to make the activities open enough to allow for mistakes and different methods of finding an answer, because then there can be (positive) disagreement. If we have taught a topic several times, we can predict which tasks can lead to good class discussions. It can be helpful to build the discussion prompts into the materials (e.g. in iblcalculus ) or they can just exist in the planning mind or a teacher manual.

Am I assigning the students tasks that are “just right” to build up to the overall concept? Are my questions flexible enough so that mistakes do occur (yes we want mistakes!) but the students won’t give up? Can a student possibly answer the questions or do they need to know what I am thinking?

Deciding on the level, number and detail of tasks is one of the most difficult parts of writing an activity. If you break the concept down too much then students will not engage in the level of inquiry that will lead to a deep understanding of the concept. They will successfully complete each task but probably not understand the bigger ideas and connections. If you break the concept down too little then you may find students become frustrated and give up on the question before reaching the level of inquiry that would lead to deep understanding.
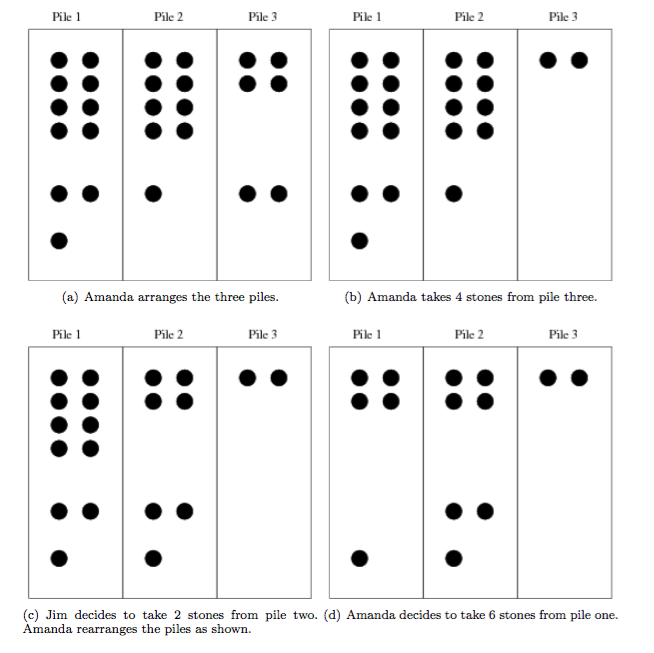
When I let my students explore the game “nim” with more than 2 piles for instance, See games book, piles activities , I know they won’t get the idea to use binary numbers (which is essential for finding the winning strategy). Most of them don’t even know what binary numbers are, and it is not very likely that they will suddenly invent them. I have tried several things to scaffold this activity:
Once I gave a mini lecture about binary numbers and let the students practice for a while until they got comfortable enough to use the idea. While this led my students to find the winning strategy eventually, they did not feel like they did it themselves. The binary numbers seemed like a strange thing invented by others and they did not own the idea. And there where no constructive mistakes either, mostly students felt that they “did not get it”.
Next time I invented an activity in which my students analyzed other students’ work (one of which used piles structured using powers of two), see picture below.



While this activity took more time, my students discovered the idea of powers of two themselves and suddenly the idea was brilliant instead of strange. Along the way, students had many incorrect conjectures (even numbers instead of powers of 2 being one of them) but used them to refine their thinking. Volker and I now also use this idea when we are working with faculty which shows that the activity automatically differentiates to different levels of knowledge.


 




Could I ask this task using a different representation? Have I developed this concept using at least two representations?

We regularly require students to work in multiple representations as a way to help them develop conceptual understanding. Multiple representations can also often help to scaffold the experience for the student so that they can develop their understanding of a particular topic through working in different representations. This also allows us to introduce topics earlier than we might otherwise have been able to. For instance let us consider the application of optimization in Calculus I. This is often left until the end of Calculus I as it is an application that is generally seen to require most of the content of Calculus I in order to approach the questions. However, simply asking these questions in a different representation can allow students to tackle these problems earlier and also develop their understanding in the process. Compare the following traditionally posed task (Option 1, slightly adapted from Hughes-Hallett, Section 4.3, Question 12) with the next task (Option 2, from iblcalculus.com):

Task (Option 1). A rectangle has one side on the x-axis and two vertices on the curve $y = \frac{1}{1+x^2}$. Find the coordinates of the vertices of the rectangle with maximum area and justify that these indeed provide a maximum area using calculus.

This task requires students to understand the meaning of the first derivative and to be able to use more advanced derivative rules to complete the problem. This necessitates that it come well into the course work. However this task could have been asked in a different representation as seen below.

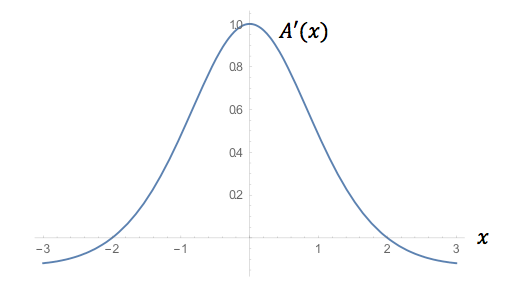
Task (Option 2). A rectangle has one side on the x-axis and two vertices on the curve y = f(x). The area of the rectangle, A, as a function of the length of the side of the rectangle on the x-axis, l, is represented by A(l) and its derivative, A′(l), is graphed below. Find the coordinates of the vertices of the rectangle with maximum area and justify that these indeed provide a maximum area using calculus.



Adapting the task to use a graphical representation instead of a symbolic one now allows the students to tackle this problem immediately after the meaning of the first derivative has been developed. This is still a difficult problem for students at this stage but with different difficulties from that of the symbolic task. This graphical task allows students to focus on interpreting information in the derivative without having to deal with the added difficulty of finding the symbolic representation for A(x) and taking the derivative symbolically. As a result this can help students develop their understanding of optimization and still allow them to return to the symbolic task later.

Questions after the writing phase

After we have finished writing the tasks we like to ask ourselves a few more questions before actually trying them out.

Have I done the tasks myself?

Maybe this sounds silly since of course we know how to do these tasks, right? However, it is interesting to see what we notice when we actually do every single prompt ourselves. How long does it take us? In how many different ways can we do the tasks? Which mistakes can we predict? Do we notice any sudden gaps in the sequence of tasks? By thinking about these questions as we work through an activity, we can be prepared for some of the good learning moments that will arise in the classroom and begin to make decisions about how we will navigate those.

What are the skills that students need to recall or develop in this activity? Are they prompted to do that appropriately?

There are many skills that we expect students will be able to draw upon when doing mathematics. It is very helpful for the students if you highlight what those skills are and make it clear whether the students are expected to bring this skill into the activity already or if it is developed in the activity itself. If it is a skill that students should have already developed either in this course or a previous course it may even be helpful to ask them to review that skill before coming to class so that they are prepared and you do not have to spend class time on that skill unnecessarily.
For instance for the speeding task from IBL Calculus 1, Module II, Course Activity 1 , the students need to understand functions and the notation f(x), linear equations, graphing lines and other functions, and making sense graphically of the slope of a line.

Is there anything in this activity that I expect students will be able to repeat in a similar setting – a method they are developing to approach a particular type of problem? If so am I providing practice opportunities for them so that they recognize this as a method? Am I highlighting this to them?

We have found that if this is not made explicit then students may always return to reasoning from the concept to recreate the method. Although in many cases we are delighted with this as it shows that students really understand what they are doing, in more complicated tasks it is helpful if students don’t have to reason through every single piece necessary to reach a solution. Instead, students can recall a method which they understand well as they have developed it through reasoning from concepts but that they do not have to repeat the reasoning each time. This allows them to focus on wrestling with bigger ideas and developing their understanding further.

Questions to ask during Revision

Did the students make mistakes?

Often we and the students learn a great deal from the mistakes students make while working on an activity. If the students made absolutely no mistakes the tasks are probably too easy and students are unlikely to be engaging in developing their own reasoning process to develop understanding of a new concept. We want there to be several paths to understanding a concept and we celebrate mistakes for the learning opportunities that they create, see also the video examples of multivariable calculus . We regularly revise our activities so as to hit the right level of mistakes in the student work. In some cases, we purposefully present the students with incorrect approaches to a problem so that they can identify mistakes and explain why the reasoning process engaged in by a hypothetical student is incorrect. This is an approach that aids students in the development of conceptual understanding.

Did I have to give a lot of hints? Did the students get frustrated?

Both giving a lot of hints and frustration often result from tasks that are too large or unwieldy for students to make any progress with. This often leads to students not making it far enough into the problem to make productive mistakes.
If this is indeed what happens then this is a sign that this particular task or sequence of tasks needs more scaffolding. A great way to introduce this scaffolding to an activity is to think about the additional questions that you posed to students in class to help them and to incorporate these into your activity. In some cases however we may have provided additional hints to some groups or there may have been some frustration on the part of students but actually many students progressed through the activity fine and developed the understanding that we were hoping for. The experience of a few students can have a major impact on our impression of a class but does not necessarily mean that any change is called for. Sometimes it is helpful after such a class to ask all students a quick exit question and base our evaluation of the tasks and activity on this exit survey from all students instead of our impression of a class.
It is also possible that we gave too many hints during class because we could not wait for the students to get there themselves. Students need to learn persistence and so we need to learn to step back. In this case the activities may be “just right” and we have to adjust our own behavior and beliefs instead of adjusting the tasks.

Did the tasks connect well with my meta-goals?

It is a lot of work to keep our meta-goals in mind at all times. But because of the importance we put on this aspect of our class we find it is worth checking back regularly to see if the confidence level is going up, if students are communicating more easily, if they dare to make mistakes and ask the risky questions, if they seem interested in mathematics in general, etc. Detecting what’s still missing in the meta-goals can hugely inform how to adapt an activity further to get at some of the more subtle learning that we are interested in.
If my students for instance still lack confidence and don’t believe in themselves enough to even start thinking, I might adapt the next activity so the first part is an “easy success”. Then the activities can continue to increase in difficulty to show students that they can actually do it and practice persistence (which goes hand in hand with confidence).
In our straight-cut origami activity ( in the games book) for instance I can let students first cut out a symmetric shape. The conjectures they make from cutting out symmetric shapes are not necessarily helpful for finding conjectures for the general case, but the “easy success” is absolutely necessary to build up enough confidence and persistence to cut out the next (much harder) shape.

What have assessment and evaluation told me about my activities?

We know a lot about our students from observing them do mathematics during class. But additional information is very helpful in learning about the exact skills, methods, understanding and meta skills students have developed.
Asking students to write up careful explanations of their reasoning for a task, completing practice problems, quizzes etc. can provide us with information about what the students actually learned. (See also our assessment page) This in turn can help us adapt the activities. Maybe students only learned the skills but not the deeper understanding? Or vice versa? I am still learning how to write assessment activities that really tell me what I need to know. For instance, I just got the idea form Mairead that leaving out the first obvious mistake as an optional answer in a multiple choice question can help students realize their mistake right away and change their thinking while they are doing the assessment. So they are learning during the assessment and I get a more refined picture of their “next” mistakes.
Surveys or journals show us students’ beliefs and attitudes toward doing mathematics. They are usually quite able to assess themselves how confident, persistent they are and what they would need to work on next.

Resources:

You can use this handout to just look at the questions (without any explanations). Please let us know if you found this blog helpful and if you know of other resources that help with writing inquiry activities.

We found the following papers very helpful in thinking about writing inquiry activities:

1) LITHNER, J. (2006). A framework for analyzing creative and imitative mathematical reasoning. Umea, Department of Mathematics and Mathemical Statistics, Umea universitet.

2) WEISS, R. Designing Problems to Promote Higher-Order Thinking. New Directions for Teaching and Learning. 2003.95 (2003): 25.